The Peloton Universe: speculations on gravitational drafting as an explanation for the dark matter problem and cosmic de-coupling as an explanation for dark energy
WARNING: Highly speculative and far-from-complete post based on a highly superficial understanding of cosmology. Most of the ideas presented here are probably wrong, but perhaps may prove of passing interest to some. If nothing else, some of the Figures may be interesting to review and consider. This is also a work in progress which I put on hold in 2018 and since I likely will not get a chance to take up again in any serious way for a while, I post it for whatever it may be worth.
_________________________________
Summary: Based on analogous terrestrial-scale processes, I propose a form of gravitational drafting in which galactic-scale masses moving through space create a drafting region or wake in which following masses increase their velocities. This provides an alternative explanation for certain observations currently explained by dark matter. Similarly, a drafting or "peloton model" provides an alternative explanation for certain observations currently explained by dark energy.
I. The dark matter problem
Five main observations are cited as evidence for dark matter. For
a summary of these, see: https://medium.com/starts-with-a-bang/five-reasons-we-think-dark-matter-exists-a122bd606ba8.
I focus my proposed framework on three of these five: the
unexpectedly high rotational velocity of matter on the galaxy peripheries,
galaxy cluster collisions, and the cosmic microwave background (CMB). I look briefly at the other two.
1. The high velocities of matter on galaxy perimeters
1. The high velocities of matter on galaxy perimeters
Rubin
(1983) and others observed that baryonic (visible, ordinary)
matter observed at galactic peripheries moves at higher velocities than
predicted by standard models; this observation is known as the flattening of
rotation velocity curves (Sanders, 2014; among others), as shown in Figure 1.
Figure 1. Extended rotation curve of M33. Top curve shows actual (flattening)
observed mass velocities, and the lower curve shows the expected velocities
based on standard Newtonian equations (from Stefania deluca
https://commons.wikimedia.org/wiki/File:M33_rotation_curve_HI.gif)
This increase in expected velocity has been explained by the
presence of an invisible halo of matter around the galactic periphery, apparently
first called dark matter by Zwicky in 1933 (Debono and Smoot, 2016). Gravitational
lensing in the regions of galactic cluster and gaseous collisions is further
evidence for dark matter (Crowe et al., 2006).
Despite many active searches, the particles that should comprise
dark matter have yet to be found (Sanders, 2014; physicsworld.com, July 2016).
Until these particles are found, alternative explanations for the existing
evidence for dark matter are reasonable. These include Milgrom’s modified
Newtonian dynamics (“MOND”) (e.g. Milgrom, 2014; for a historical perspective
and discussion of other proposed modifications to Newtonian dynamics, see
Sanders, 2014), and more recently the “emergent gravity” theory of Verlinde
(2017).
There are several criticisms of MOND (DeBono and Smoot, 2016). Two
major ones are its failure to predict inhomogeneity and structure in the
universe, which is well accounted for by a dark matter model (Dodelson, 2011);
and the apparent passage of dark matter through baryonic matter during high
velocity galaxy cluster collisions, such as has been proposed to occur for the
Bullet Cluster (Clowe et al., 2006). See this presentation for an overview of how baryonic acoustic
oscillations and gravitational lensing lead to the dark matter hypothesis: http://slideplayer.com/slide/6878195/
II. A novel conceptual framework
1.2 Gravitational drafting and dark energy
The second general suggestion, consistent with the first, is that dark energy, insofar as it is currently required to explain the observed rapid expansion of the universe about 5 billion years ago, may be explained by a phenomenon in which leading and following (drafting) baryonic matter, on a cosmic scale, de-coupled its drafting interactivity at a critical density in the expansion history of the universe. In this conception, such a “cosmic de-coupling event” caused an epoch of accelerated expansion between regions of matter that were previously coupled by gravitational drafting. In this model, drafting matter actually decelerates in relation to the leading matter, but the observed effect is an accelerating expansion of the distance between de-coupled matter.
II. A novel conceptual framework
I propose that a “cosmological drafting” model to reconcile these problems. I do not propose a mathematical model that can account for my proposed cosmological drafting framework and I confess my own limitations in mathematics are such that I am largely incapable of producing a mathematical model to support my proposal. Nonetheless, what follows are some ideas which perhaps brighter minds than mine will find interesting enough to test against existing data and mathematical models.
1. Gravitational drafting
In this conception, “energy saving” is synonymous with an effective increase in the gravitational force that causes matter to travel at higher velocities than it would in the absence of this energy-saving effect. The proposed effect is analogous to aerodynamic and hydrodynamic drafting in which masses in motion reduce energy expenditure, and effectively increase their velocities when trailing in optimum positions relative to leading bodies, I refer to this as a “gravitational drafting” effect generated in the trailing space behind matter in motion at critical velocities.
On the other hand, the effect is unlike aerodynamic and hydrodynamic drafting because there seems to be no drag force in space that slows the leading matter. But the proposal remains that space is reconfigured in the trailing regions behind matter moving through empty space, such that a wake region is generated.
On the other hand, the effect is unlike aerodynamic and hydrodynamic drafting because there seems to be no drag force in space that slows the leading matter. But the proposal remains that space is reconfigured in the trailing regions behind matter moving through empty space, such that a wake region is generated.
1.1 Gravitational drafting and dark matter
In this conception, dark matter need not be present to account for certain cosmological observations, as prevailing theory demands. Rather, the implication is that gravitational drafting is a property of space that emerges in a region of space that trails behind matter as it moves through space at critical velocities or energetic intensities, and is not a property of matter per se. I propose that cosmological observations otherwise explained by dark matter may be explained as gravitational drafting. I suggest this drafting effect may be indicated by an alternative interpretation of available gravitational lensing images, which I explore in this post.
1.2 Gravitational drafting and dark energy
1.3 Proposition: the noted increase in galactic velocities is due to a velocity equalization effect when matter trails other matter at
comparatively high velocity inside gravitational wake regions
I propose that the motion of mass through space creates a kind of
gravitational trailing wake that acts to magnify the effective force of
gravity, thus equalizing the velocities of trailing matter within the wake
region and permitting trailing matter to travel at approximately equal speeds
to leading massive objects. The proposed
phenomenon is like aerodynamic or hydrodynamic drafting in which drag is
reduced in trailing wake regions behind bodies in motion, commonly observed in earth-scale systems.
The closest published theory to my proposition that I have found in the
literature is that of Furlanettor and Loeb (2001), who proposed the existence
of “hydrodynamic” gravitational wakes with a cone-like structure. In their paper, the authors describe the wake
structure in gaseous matter (a “collisional medium”) and dark matter (a
“collisionless medium”) as matter moves through space, but they do not appear
to suggest that wakes emerge as a property or structure of empty space.
My proposition goes a step farther to suggest that the movement of matter through space generates a gravitational wake in the structure of the space itself behind the moving object (hereafter “space wake” or “drafting wake”). Trailing behind the baryonic (ordinary) matter in its direction of motion, the space wake creates an effective increase in gravitational force on matter that follows within the boundaries of the drafting (conical) wake, analogous to aerodynamic and hydrodynamic drafting regions that trail objects moving through fluid mediums.
My proposition goes a step farther to suggest that the movement of matter through space generates a gravitational wake in the structure of the space itself behind the moving object (hereafter “space wake” or “drafting wake”). Trailing behind the baryonic (ordinary) matter in its direction of motion, the space wake creates an effective increase in gravitational force on matter that follows within the boundaries of the drafting (conical) wake, analogous to aerodynamic and hydrodynamic drafting regions that trail objects moving through fluid mediums.
This effective increase in gravitational force accounts for the
flattened spiral galaxy rotational curves. The proposed presence of space wakes
and the increase in velocities facilitated by gravitational drafting seems to
be consistent with Milgrom’s MOND formulation, which effectively extends the
force of gravity over greater distances in low gravitational acceleration
circumstances, such as found at the peripheries of spiral galaxies (Sanders,
2014; physicsworld.com July 2016). In turn this increases the mean velocities
of masses influenced by gravity.
I suggest this is equivalent to a drafting or shielding effect,
which slows the deceleration rate for a following mass and can also generate a
suction force (e.g. Wang et al, 2014, in the context of drafting spheres). In
the absence of the shielding mass ahead, the trailing mass would be exposed to
higher effective-drag forces and therefore slower speeds or a higher energetic requirement to
sustain the given speed; in other words, the speed of the following mass is
sustained for longer at lower energetic output.
Thus, the proposed drafting wake effect, I suggest, is consistent
with MOND at least in the range of low accelerations where MOND works, and does
not require dark matter to explain the observed flattened rotational curves. Of
course, the big questions are therefore how and why should a moving object
through empty space generate a drafting wake region, and why should that region
behave as though it were a massive object that increases gravity. This seems to imply that the shape of space ahead of a moving mass is different from its shape in the trailing wake region, and that somehow in a way for which I make no suggestions, there is greater effective "drag" ahead of a moving mass, and a corresponding wake region behind it. Perhaps it is at this simple juncture that my ideas fail miserably.
Further, why should a high density interior region of the galaxy not exhibit the drafting effect; i.e. since the quantity of matter in the interior accurately accounts for the observed mass velocities without requiring either dark matter or modified gravity equations. For this, however, I do make a proposition.
Further, why should a high density interior region of the galaxy not exhibit the drafting effect; i.e. since the quantity of matter in the interior accurately accounts for the observed mass velocities without requiring either dark matter or modified gravity equations. For this, however, I do make a proposition.
1.4 Enter Verlinde
Erik Verlinde’s recent (2017) paper “Emergent Gravity and the Dark
Universe” may shed some light on these questions. I do not possess the
theoretical or analytical tools to offer any reasonable evaluation of his work
to determine its consistency with a gravitational drafting effect. However, I can reasonably ascertain certain
general aspects of Verlinde’s paper, including that he applies his theory to an
idealized “spherically symmetrical” (p.38, arXiv version) dark matter
equivalent displacement region of space; and that for his theoretical
framework, the topology or shape of this region is not important. This suggests that it may be possible
to deform Verlinde’s spherical region into the conical drafting region I
propose, and that this would be one means of testing both his theoretical
framework and the validity of my own propositions.
It is also reasonably clear that Verlinde’s theory involves
a volume of baryonic matter space displacement such that the displaced region
resides in a kind of “pressureless fluid” (p. 44); and that such a displaced
region contains a gravitational force equivalent. In my highly simplistic understanding, this
is just what a drafting region is: a region of volume displacement which
permits an increase, or equalization, of the velocities of bodies that follow
in the drafting region. Verlinde says
(p. 15):
“The fact
that matter causes a displacement of the dark energy medium implies that the
medium also carries a reaction force on the matter. That magnitude of this
elastic force is determined in terms of a residual elastic strain and
stress. We propose that this force leads
to the excess gravity that is currently attributed to dark matter.”
The elastic force seems to be analogous to the “suction” force of
drafting, and/or the reduction in drag force that comprises drafting. Of
course, with drafting, a leading body provides a shielding effect from drag,
which is reduced for the following mass. Verlinde does not speak of drag forces
in space, but it seems a reasonable hypothesis that the motion of matter
through space perturbs and displaces space conically in a way that generates
increased equivalent gravity.
1.5 Drafting at the terrestrial scale
To illustrate my proposition, let’s look a little more closely at
certain basic principles of aerodynamic and hydrodynamic drafting.
a. Aerodynamic context
First, the energy saving effect of drafting increases with the
size of the leading mass. For example, one cyclist drafting another at 40 km/h
saves about 26%, in a no-ambient wind condition; one cyclist drafting two
others in single files saves about 27%, while a cyclist in a group of eight,
saves about 39%; a cyclist drafting a large box-truck saves about 62%, as shown
in Figure 2 (Hagberg and McCole, 1990). Clearly
a small object drafting a much larger one saves more energy.
Figure 2.
Energy saving among cyclists in different configurations (from Burke,
1995, Figure 10.3; as adapted from Hagberg and McCole, 1990).
Secondly, the magnitude of drafting diminishes as function of distance (Olds, 1998). See Figure 3, with reference to Olds (1998) equation 1 and adapting his Figure 1. This models the diminishing drafting benefit as function of distance between cyclists up to about 3 m when the drafting quantity approaches zero.
Figure 3. Reduction of drafting benefit as a
function of distance between cyclists (correction factor from Olds (1998) eq.
(1), and corresponds to increasing drag). Rapidly accelerating loss of drafting
benefit between about 1.5 m and 3.0 m, corresponds to increased power
requirements to maintain the same speed due to increasing drag force as wheel
space increases. Adapted from Olds (1998), Fig 1.
A third important principle
of drafting is that its magnitude increases with velocity; for example, below
about 16 km/h, there is no drafting benefit for following cyclists (Kyle,
1979). At cosmic scales, this suggests
there may be a threshold velocity above which the drafting effect becomes
observable. The function may be derivable by a simple analysis of the
rotational curves, as shown in Figure 1. By simplified approximation, according
to Figure 1, the threshold mass velocity at which the gravitational drafting
effect emerges appears to be about 40 km/s, and the effect increases as a
function of mass velocity thereafter. Indeed,
ultra-compact dwarf galaxies with velocity dispersion between 24 to 37 km/s,
have been shown not to contain dark matter (Scarpa, 2006; Drinkwater et al.,
2003); this suggests the gravitational drafting effect emerges at mass
velocities greater than 37 km/s.
If a simple velocity function and threshold is insufficient, I
suggest that in addition to mass velocities, the drafting component should be
considered along with the density of the mass distribution, and the relative
magnitudes (and possibly their volume) of the masses that are coupled by
gravitational drafting. Thus, I suggest an equation that models the
relationship between these factors will describe matter velocities in high
density regions in the interior regions of the galaxies (Scarpa, 2006), and
scales proportionately to decreasing matter densities toward galaxy
peripheries, such that the
drafting effect is small in high density regions (and/or below the velocity
threshold), and large in low density or small acceleration regions. To
avoid the criticism that I am constructing some arbitrary function without
grounding it in physical principles, I will endeavor to show why in principle this relationship can work.
b. Hydrodynamic context
b. Hydrodynamic context
In the hydrodynamic context, Hoerner (1965) describes the drafting
effect:
“Shielding
Effect. In
case of two bodies placed one behind the other, the drag of the second one is
usually smaller than in free flow, because of reduced dynamic pressure within
the wake of the first body. In the example presented in figure 1, the
drag of the second disk is even negative, up to a distance of more than 2
diameters, evidently because of suction behind the first plate. As the distance
between the two disks is increased, the drag of the second one gradually
approaches the value known under free-flow conditions (Cd = l .17). This type
of shielding effect can have some consequences in motorcar racing, where a
competitor may run for a while within the wake of another car ahead of him.”
Similar to the
reduction of drafting effect in the aerodynamic context shown in Figure 3,
Hoerner (1965, p. 8-1, Fig 1) indicates that the hydrodynamic drafting benefit
diminishes with the distance between the lead and drafting cylinder, and
becomes zero at a distance of about 7 diameters, when for the following
cylinder Cd = 1.17. Igarashi (1981)
reported the negative drag or suction force to be Cd = -0.65 up to the noted ~2
diameter range, while the reduced drag for such a downstream cylinder beyond
the suction zone to be Cd = 0.45.
Like the increased energy saving for a cyclist drafting several
cyclists or a large box-truck, a similar phenomenon is shown among “drafting,
kissing, and tumbling” spheres in a liquid medium (Wang et al., 2014; Liao et
al, 2015). When a small sphere and a
larger sphere, initially separated by some given distance, are dropped
simultaneously under gravity in liquid, the small sphere will catch-up to the
large sphere and travel at about the same velocity as the larger sphere. In
this configuration, the smaller sphere will travel faster in the drafting
region of the larger sphere than it -- the smaller sphere -- would if dropped
with a sphere of the same size, or if the initial starting configuration was
reversed (i.e. the large sphere followed the small), (Liao et al. 2015), as
shown in Figure 4 (from Liao et al., 2015).
Figure 4. From Liao et al., 2015. In Setup-C, the
small sphere falls faster if it trails a larger sphere.
Liao et al. (2015) confirm the finding of Wang et al. (2014), who describe the process (at p. 31) as indicated in Figure 4 above, and as shown in Figure 5, below:
“The reason
for this behavior is that there is a strong suction effect on the smaller
(trailing) particle due to the low pressure in the wake of the larger (leading)
one, which causes the upper particle to fall more rapidly than the lower one…As
shown in Figure 16, the wake flow behind the larger particle extends beyond the
smaller one, indicating that the smaller particle is strongly influenced by the
wake of the larger one, and the smaller experiences less drag and thus it
sediments at higher velocity than the larger one.”
Figure 5.
Trailing wake behind larger and smaller spheres (from Wang et. 2014, Fig.
16).
Extrapolating from Figure 5, we can see that a greater large/small sphere ratio could permit several small spheres to fill the wake space behind it. Thus, a high density region of spheres reduces the wake space. I will later expand on the importance of this in the context of dark matter.
Note that the spheres and wake regions in Figure 5 are flattened
in two dimensions. Since the drafting
events occur in a fluid medium, we would expect the wake to be three-dimensional:
an elliptical cone.
Figure 6. Elliptical cone (S. Cheng; retrieved
online, July 18, 2017).
1.6 Galactic cluster collisions
1.6 Galactic cluster collisions
The second major source of evidence for dark matter is high velocity galaxy cluster collisions (Markevitch, 2004; Clowe, 2006).
Keeping certain basic principles of aerodynamic and hydrodynamic drafting in mind, let’s look at some of the images of galactic collisions, and their stated dark matter regions.
Keeping certain basic principles of aerodynamic and hydrodynamic drafting in mind, let’s look at some of the images of galactic collisions, and their stated dark matter regions.
The Bullet Cluster:
Figure 7.
Bullet cluster collision (from Chandra Harvard online).
As indicated by Figure 7, under the currently leading paradigm,
dark matter (blue) has passed through the gaseous ordinary matter (pink) so
that the dark matter has shifted to a location in front of the ordinary matter,
in the direction of motion (i.e. blue portions in the image have passed from central regions through the pink to the exterior regions).
Here is a video that illustrates the prevailing dark matter
hypothesis for the bullet cluster collision:
https://www.youtube.com/watch?v=eC5LwjsgI4I
This is said to be possible because the velocity of the gaseous
matter is reduced by a drag phenomenon, thus allowing the frictionless dark
matter to pass through to the ordinary matter so that it resides ahead (to the exterior, in Figure 7) of the
baryonic matter.
1.7 The Bullet Cluster viewed
under the “peloton model"
Is there at least one other possible interpretation of this image?
What if the dark matter is not matter at
all, but is instead a kind of gravitational drafting wake phenomenon, such as
observed in pelotons and many other biological and non-biological collectives (for
a overview of energy saving mechanisms in nature, see our a review Trenchard
and Perc, 2016)? Such a model does not require the presence of dark matter, but
instead is equivalent to an increased gravitational force that trails the
ordinary matter as it moves at enormous velocities through space.
In this model, like the aerodynamic and hydrodynamic drafting effects described above, the motion of ordinary matter through space creates a kind of low-pressure fluid wake effect in which the matter that follows in the wake region can, within a certain critical distance, sustain equal speed to the matter ahead. The effect diminishes with distance, just as we would expect from standard Newtonian inverse square law for gravitational effect, and in turn Milgrom’s modified version thereof (Milgrom, 2014; which I will discuss more further). So we should observe strong gravitational drafting closest to the leading mass, and a threshold distance at which the following matter lags outside the gravitational drafting, thus de-coupling from the leading mass, and advancing at its own intrinsic velocity.
In this model, like the aerodynamic and hydrodynamic drafting effects described above, the motion of ordinary matter through space creates a kind of low-pressure fluid wake effect in which the matter that follows in the wake region can, within a certain critical distance, sustain equal speed to the matter ahead. The effect diminishes with distance, just as we would expect from standard Newtonian inverse square law for gravitational effect, and in turn Milgrom’s modified version thereof (Milgrom, 2014; which I will discuss more further). So we should observe strong gravitational drafting closest to the leading mass, and a threshold distance at which the following matter lags outside the gravitational drafting, thus de-coupling from the leading mass, and advancing at its own intrinsic velocity.
I suggest that this de-coupling effect also has implications for
the presence of dark energy, which I will discuss more in paragraphs that follow. I suggest that this drafting effect, because
it has the same effect as gravity in that it permits matter to travel through
the drafting region faster than it would in an un-coupled state, distorts space
in much the same way as ordinary matter, and therefore can be observed by
gravitational lensing.
The analogous circumstance may be present in star and galaxy
clusters. The standard Newtonian equation shows that gravitational force
diminishes as the square of the distance. A drafting effect might
simply modify the gravitational effect as a function of the nearness of other
visible matter such that matter creates an additional "gravitational
wake" in a region behind the moving mass (i.e. on the opposite side of the
mass to its direction of motion in space). This predicts therefore that
there is an increased effective gravitational force in the region behind the
star, star cluster, or galaxy cluster. On the side of the star that faces
the direction of movement, the prediction is that the standard Newtonian or
Einsteinian gravitational forces exist. The magnitude of the "wake"
diminishes with distance to some unknown threshold distance, beyond which there
is no drafting effect.
A two-dimensional drafting wake trails in an approximate triangle
from the leading object, as shown in Figure 3, so it is reasonable to expect a
three-dimensional wake in cosmic space to be an elliptical cone, as shown in
Figure 4. In a rotating spiral galaxy, for example, the hypothesis here is that
passing stars will capture following stars in its conical wake and, in effect,
pull them along at velocities that are faster than expected by the standard
Newtonian equation.
Therefore, applying what I refer to as a peloton model with
approximate elliptical gravitational drafting cones, I suggest the images of the Bullet Cluster collision can
be mapped like these:
A.
B.
Figure 8A. Left cluster wake cone. Apparent dark matter (blue) trails leading matter in
a conical, expanding drafting wake, as ordinary matter moves approximately to
the right. B. Right cluster wake
cone. Apparent dark matter trails in conical wake behind ordinary matter which
moves approximately down left and toward the viewer (all cone drawings by HT). Whether the nose of the
cone is peaked or flat is difficult to ascertain from the image
Figure 9. Intersecting wake cones during collision.
Figure 9. Intersecting wake cones during collision.
In this interpretation, we can see regions where we would expect to see more blue imaging if the drafting wakes were uniform. This is a weakness of the proposed model, but perhaps there are discrepancies in the data, effects of other stellar bodies, or space gaps in which there is no ordinary matter around which gravitational lensing might be observed, among other things. Regardless, I suggest that the high density blue areas shown to the right of the nose of the left cone, and to the left of the right cone, are misleadingly small, and that the gravitational wake region is better represented as conical, as indicated.
Under this model, no discrepancy arises by the intersection of the
ordinary matter on the non-matter drafting region behind each cluster, because
the matter can fill the wake region without any difficulties in principle. Also, we should observe gravitational lensing
in the entire conical wake region, even those parts that are dominated by
gaseous or other matter, and this does appear to be the case. If this is not the case, then this would be a
clear weakness of the model.
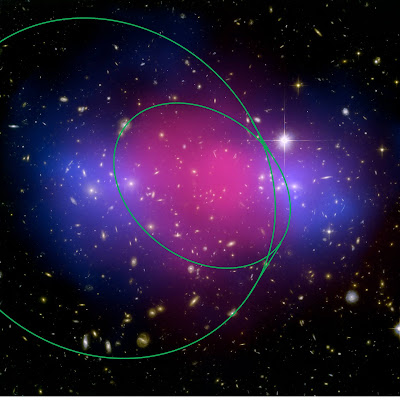
1.8 El Gordo collisions
Figure 10. El Gordo showing apparent dark matter regions in blue, from Chandra’s x-ray observatory (https://chandra.harvard.edu/photo/2014/elgordo/).
“As with the Bullet Cluster, there is evidence that normal matter,
mainly composed of hot, X-ray bright gas, has been wrenched apart from the dark
matter in El Gordo. The hot gas in each cluster was slowed down by the
collision, but the dark matter was not.”
Now, looking again at the El Gordo collision, and consider my alternative hypothesis that apparent dark matter
trails in a cone behind the ordinary matter:
Figure 11A. Left cluster cone moving approximately from left to right. Nose of cone indicates high density region of ordinary matter. Apparent dark matter “drafting zone” encompasses enormous region of space in a three-dimensional cone, but trails behind the high density ordinary matter, and does not pass through the ordinary matter as weakly interacting particles. B. Right side cluster cone moving from right to left. Apparent dark matter, or what I refer to as a drafting zone or region, encompasses an enormous volume of space, apparently expanding outward toward the viewer.
Figure 12. Intersecting
right and left cones of the El Gordo collision. If the right cluster (yellow)
contains more mass and/or expands into space toward the viewer, it makes sense
the cone would either be larger or appear to be larger. Similarly, the nose of
the cone would be smaller because it is farther away, on the far side of the
combined mass.
1.9 MACS J0025
Figure 13. MACS J005 (from Chandra Harvard, online)
In the MACS collision, the matter from the colliding galaxies has been described as having “pooled in the middle” http://spacetelescope.org/images/heic0818a/ as also indicated by the red and green contour map shown in their Figure 9 (Harvey et al. 2015).
I have not found a reference that shows where the highest densities of the gaseous and galactic matter occur for the two colliding masses. So, in approximating the drafting cones, there is more guesswork for MACS J0025 than for Bullet Cluster and El Gordo. But if we view the collision between matter fronts as one gaseous/galactic mass going roughly into the page away from the viewer, and one approximately coming towards the viewer, we can reasonably infer the overlapping conical regions as follows:
Figure 14A. Left cone, expanding away from view,
meaning gaseous/galactic mass approaches from farther out. B. Right cone, apparently expanding toward us, with direction or
motion into the page. Perspectives permit reversed approaches.
Figure 15.
Intersecting left and right cones for the MACS J0025 collision. Collision
appear to be nearly head-on but into and away from the given view. This results
in overlapping cones. Thus, it appears that the left drafting cone (green) is
expanding away from view, while the larger visible mass region is closer to us,
on this side of the image; the right cone is expanding toward us, with the
smaller visible matter region is on the far side of the image. It is however, not obvious what regions
comprise the matter for each drafting cone.
At present, I have not looked closely at images of the more complex Abell and “Trainwreck” collisions (or others) and their dark matter regions to ascertain where the wake cones might be.
1.9 Some thoughts on Markevitch’s dark matter area derivations
The Markevitch et al (2004) and Clowe et al. (2004) papers and
their descriptions of the gravitational lensing effects around the Bullet
Clusters are the basis for one of the strongest pieces of empirical evidence in
favor of the existence of dark matter. For instance, Sean Carroll is emphatic
that the Bullet Cluster proves the presence of dark matter: https://www.youtube.com/watch?v=4uogQiH5Yx4.
Far be it for me to be in any position to criticize the approach
taken by Markevitch et al. (2004), but there appear to be aspects of their
approach that one might reasonably question.
With respect to their weak lensing measurements they say they use a King
mass profile to fit the lensing signal to determine the total masses of the
subcluster and the main galaxy clusters.
A King mass profile appears relatively straightforward, involving three
main paramaters: 1. Central brightness; 2 Core radius, and
3. Tidal radius, the point at which brightness vanishes: http://www.astro.caltech.edu/~aam/science/thesis/total/node20.html
The authors define the main “cluster” as comprising the region to
the left of the bullet-shaped cluster, and bullet “subcluster” as the obvious
region to the right. They state:
“The subcluster
mass signal is detected to r ≃ 150 - 200 kpc from the
mass (or galaxy) peak, beyond which the subcluster may be tidally stripped (C04)
... For the sake of modeling, we will adopt a King profile with rc
= 70 kpc and ρ0 = 1.3×10-24 gcm-3, truncated at rtr = 150 kpc, which
adequately describes the lensing data.”
Figure 1 from the Crowe et al.
(2004) paper indicates the 200 kpc white bar (copied below):
Figure 16. Image from Crowe et al. (2006) indicating
the white bar is 200 kpc at the distance of the cluster.
The authors appear to arbitrarily constrain the
cross-sectional area of what they believe is a dark matter region. This is because the authors appear to have truncated the tidal radius at 150
kpc (as quoted above) when there apparently exists a wider lensing area -- apparent from
the faint area of lensing at the top of top right-of-center region in Figure
11, and more easily seen in Figures 2 and 3, and a view by the naked eye
of lensing phenomena.
I confess my analysis here is weak, and needs to be considered a lot more carefully. However, my point is that perhaps the data could be re-analyzed in the context of a drafting wake model.
I confess my analysis here is weak, and needs to be considered a lot more carefully. However, my point is that perhaps the data could be re-analyzed in the context of a drafting wake model.
1.10 The peloton model appears to
be consistent with Milgrom’s modified Newtonian dynamics (MOND)
Under
Milgrom’s MOND, dark matter is not a required explanation for the increased
velocity of matter observed at galactic extremities (Milgrom, 2014; also see
historical review by Sanders, 2014).
Thus, below a certain threshold gravitational acceleration threshold,
found largely in lower density galactic regions (Famey, 2005; Scapra, 2006), a
modified Newtonian gravitational equation produces predictions that accord well
with empirical data (see Sanders, 2014).
1.11 How does this fit a peloton model?
First, consider some images:
First, consider some images:
Figure
16A. Compact, high density, peloton, at comparatively low speed. Drafting
regions are completely filled, except for peripheral riders. B. Single file, low density, peloton. All riders
are riding at or near maximal sustainable outputs and so the speed of this peloton is expected to be considerably higher than in Figure A. Partial drafting regions to the
sides of each cyclist are not filled, but at these outputs, riders must ride in optimal
drafting position or be dropped. C.
Mixed compact-single file peloton (image credits in references).
Is
the high density interior region of galaxies akin to that of Figure A, in which the total
drafting area is generally filled (except on peripheries) and in which there
are high acceleration gradients between cyclists because average speeds are slower? Similarly, is the galactic exterior or periphery akin to the high energy output phase shown in B, in which the acceleration
gradients between cyclists are small because the speeds are higher, but where drafting cones are only partially filled? Is
an entire galaxy something akin to what we see in C, where there is a high
density, lower speed, interior region; and a low density, higher speed, exterior? The analogy suggests that the passing or acceleration gradient, which is greater in the interior regions of the peloton and smaller at peripheries, is important; i.e. the proposal is that these different acceleration gradients according to density and relative energetic outputs may offer some explanatory power for the MOND equations which do not hold in high density interior galactic regions where the proposed drafting cones are mostly
filled, but does apply in peripheral galactic regions where there are
greater regions or volumes of unfilled drafting cones.
III. Dark energy: cosmic de-coupling
It has also been proposed that the universe has been undergoing an
accelerated rate of expansion, since about 5 billion years ago. This expansion has been attributed to dark
energy comprising some energetic principles that have not yet been empirically
established.
Thus, when the
distance between the two cylinders increases, a critical point is reached when
the drag for the downstream cylinder is the same as for the upstream one. The effect is the same for cyclists, and can
be illustrated by a simple plot, based on Olds’ (1998) equation (1). The magnitude of drafting benefit diminishes
to zero as the distance between cyclists increases, up to about 3 m. If a
cyclist keeps his front wheel within a meter of the rear wheel of the cyclist
ahead, there is negligible loss in drafting benefit. After 2 m, the reduction in benefit
accelerates up to 3 m, when drag force for the following rider is the same as
for the leader (assuming the same velocity and other drag parameters), as shown
in Figure 11.
If the following
cyclist is weaker than the leading cyclist, and can only sustain the speed of
the leader by riding in the optimal drafting zone up to 1m, and if she happens
to drift backward to a wheel spacing between 2m and 3m, her speed will fall
rapidly even at the same power applied in the optimal drafting zone, and wheel
spacing will increase suddenly. This is because the rapid onset of increase
drag between her and the cyclist ahead will cause her to decelerate. This rapid deceleration is a
de-coupling event in which the following cyclist falls outside the drafting
zone of the lead cyclist.
Consider cyclist A whose maximum sustainable
power (MSP) is 300W; and cyclist B whose MSP is 400W. By exploiting the
drafting zone behind cyclist B, A may sustain the speed of B at ~43 kmh by
drafting, but A is otherwise capable of sustaining only about 38 kmh when
cycling in isolation on his own. If the wheel
spacing between A and B approaches 3m (or drifts laterally beyond the optimal
drafting angle), his speed will suddenly decelerate to ~38 kmh even while
sustaining 300W (using: http://www.cyclingpowerlab.com/PowerSpeedScenarios.aspx). This deceleration upon de-coupling results in
a rapid and accelerated expansion of the distance between them.
I postulate that an
analogous cosmic de-coupling effect may be responsible for the dark energy
accelerating expansion of the universe. The universe expanded at a constant rate until
about 9 billion years ago. The accelerated expansion of the universe that began
about 5 billion years ago need not be accounted for by dark energy, but is merely
the expected effect of de-coupling when the distance between cosmic matter in
the universe generally reached a critical quantity.
Olds (1998)
provides a similar equation in the context of drafting cyclists, in which the
drafting magnitude diminishes with distance between the rear wheel of the
leading cyclist and the front wheel of the following (drafting) cyclist up to
3m, when the drafting effect is zero and the drag force is the same for the
front rider and the following cyclist.
This model predicts several cosmological dynamics summarized as follows:
Predicted dynamic
|
Evidence
|
There is greater “drafting” or energy saving capacity in higher
density clusters (1), because the drafting cones are additive and filled.
Tchieu, A.A.,
Crowdy, D. and Leonard, A., 2010. Fluid-structure interaction of two bodies
in an inviscid fluid. Physics of Fluids, 22(10),
p.107101.
|
High density galactic clusters exhibit higher density dark
matter than lower density clusters of roughly equal mass, as explained:
and:
https://physics.aps.org/articles/v9/9 based on Miyatake, H., More, S., Takada, M.,
Spergel, D.N., Mandelbaum, R., Rykoff, E.S. and Rozo, E., 2016. Evidence of
halo assembly bias in massive clusters. Physical review letters, 116(4),
p.041301.
|
As the corollary of the above, drafting capacity diminishes as
cluster density falls.
|
|
When drafting bodies de-couple there is an accelerated increase
in distance between them, which may appear as the accelerated expansion of
space between drafting bodies.
For cyclists see:
Olds, T., 1998. The mathematics of breaking
away and chasing in cycling. European journal of applied physiology
and occupational physiology, 77(6), pp.492-497.
For the equivalent hydrodynamic effect
between a downstream drafting cylinder, see:
|
The threshold point that occurred ~5 billion years ago, when the
expansion of the universe began to accelerate, may be the de-coupling point
when following ordinary matter exceeded the boundary of the gravitational
wake or energy saving region. This dynamic could therefore negate the need
for dark energy. Also, the de-coupling
period or region might show a gradual acceleration in expansion as the
coupling “elastic” approaches the final and complete point of
de-coupling. It would depend on what
part of the expansion phase we are in and able to observe. If the universe is
currently still in the “snapped-elastic” phase of cosmic expansion, we should
observe a high rate of accelerated expansion. At some point, however, the
“wake model” predicts stabilization of the expansion rate.
|
Once the “cosmic de-coupling event” (CDE) occurred, apparent expansion
accelerates rapidly for a period, and then the rate of accelerated expansion
decays to zero (expansion stabilizes) and cosmic bodies (and clusters) then proceed
at their own intrinsic velocities and expansion at a stable rate continues.
So, the prediction is that the rate of accelerating expansion
will not be the same, depending on how far into the past you look. Thus at
different periods , you may find a) a rapid accelerating expansion phase; b)
a falling rate of accelerating expansion phase c) a stable expansion phase.
All phases comprise CDE which ends in a rate of stable expansion.
The duration of CDE should be calculable. Applying the aero and
hydrodynamic analogies, CDE should occur over a comparatively short duration. Under this
model we may predict that nearby cosmic bodies exhibit more stable expansion
rates, whereas ones observable in time approaching 5 billion years in the
past should show higher accelerated expansion.
|
Even if there was not a generalized CDE, this model could
account for differences in measured rates of expansion in different parts of
the universe (Racz, et al., 2017; see also: Tian, S. et al., 2017.)
Nielsen, J.T., Guffanti, A. and Sarkar, S.,
2016. Marginal evidence for cosmic acceleration from Type Ia
supernovae. Scientific reports, 6, p.35596.
Further evidence would be delineation of differential cosmic
expansion rates as a function of how far back in time the expansion is
measured.
|
There should be some detectable gravitational wake exhibited by
smaller mass objects, like the sun or planets, resulting in increased
velocity.
|
Anomalous, periodic velocities have been observed in satellite
flybys at certain latitudes (Anderson et al., 2008). Perhaps these can be partly
attributable to a small-effect earth drafting wake region, or one combined
with a solar wake region.
|
There are optimal drafting positions in which velocity of
following mass is greater than in other positions. By analogy, for cyclists,
if there is no side wind, the optimal zone is directly behind the rider in
front and as close to the rider as possible, with some increased energy
saving among a group up to eight cyclists; for fish the zone is at angles to
the leading fish since turbulence directly behind reduces energy saving, as
it is for birds.
|
Unknown, although existing data may indicate whether magnitude
of the proposed gravitational wake diminishes with distance.
|
The drafting cone varies in size according to the mass of the
matter it trails, with some relationship to the volume of the matter, and not
just its mass.
|
Kim et al. (2016) have indicated that dark matter likely
oscillates around the centre of the visible mass for billions of years. This
is consistent with a trailing cone where there is no separation between dark
matter and visible matter, but will trail behind the visible matter in the
direction of motion. Any change in trajectory, particularly an elliptical
trajectory, will move the position of the trailing cone, and it will change
size depending on velocity and whether the matter clumps with other matter.
Under the prevailing view matter is weakly interacting with visible matter,
but can move though visible matter, which suggests the possibility that it
can separate from visible matter.
|
_______________________
Anderson, J.D.,
Campbell, J.K., Ekelund, J.E., Ellis, J. and Jordan, J.F., 2008. Anomalous
orbital-energy changes observed during spacecraft flybys of Earth. Physical
Review Letters, 100(9), p.091102.
Bradač, M.,
Allen, S.W., Treu, T., Ebeling, H., Massey, R., Morris, R.G., Von Der Linden,
A. and Applegate, D., 2008. Revealing the properties of Dark Matter in the
merging cluster MACS J0025. 4–1222. The Astrophysical Journal, 687(2), p.959.
Burke, E. 1995.
Serious Cycling. Human Kinetics. Champaign, Ill.
Clowe, D.,
Gonzalez, A. and Markevitch, M., 2004. Weak-lensing mass reconstruction of the
interacting cluster 1E 0657–558: Direct evidence for the existence of dark
matter. The Astrophysical Journal, 604(2), p.596.
Clowe, D.,
Bradač, M., Gonzalez, A.H., Markevitch, M., Randall, S.W., Jones, C. and
Zaritsky, D., 2006. A direct empirical proof of the existence of dark
matter. The Astrophysical Journal Letters, 648(2),
p.L109.
Debono, I. and
Smoot, G.F., 2016. General relativity and cosmology: Unsolved questions and
future directions. Universe, 2(4), p.23.
Drinkwater,
M.J., Gregg, M.D., Hilker, M., Phillipps, S., Ferguson, H.C., Jones, J.B.,
Bekki, K. and Couch, W.J., 2003. A class of compact dwarf galaxies from disruptive
processes in galaxy clusters. Nature, 423(astro-ph/0306026),
pp.519-521.
Dodelson, S. The real
problem with MOND. Int. J. Mod. Phys. D 2011, 20, 2749–2753.
Famaey, B. and
Binney, J., 2005. Modified newtonian dynamics in the milky way. Monthly
Notices of the Royal Astronomical Society, 363(2), pp.603-608.
Furlanetto, S.R. and Loeb,
A., 2002. Constraining the collisional nature of the dark matter through
observations of gravitational wakes. The Astrophysical Journal, 565(2),
p.854. http://iopscience.iop.org/article/10.1086/324693/meta
Hagberg, J.,
McCole, S. 1990. The Effect of Drafting and Aerodynamic Equipment on Energy
Expenditure During Cycling. Cycling Science Publications, as adapted by Burke,
E. 1995. Serious Cycling. Human Kinetics. Champaign, Ill. 261 pages, p. 193.
Harvey, D.,
Massey, R., Kitching, T., Taylor, A. and Tittley, E., 2015. The
nongravitational interactions of dark matter in colliding galaxy clusters. Science, 347 (6229),1462-1465.
Hoerner,
S.F. 1965. Fluid-dynamic drag: practical
information on aerodynamic drag and hydrodynamic resistance. Hoerner Fluid
Dynamics, New Jersey, 455 pp. ch. 8 Interference Drag, p. 8-1.
IGARASHI,
T. 1981. Characteristics of the flow around two circular cylinders arranged in
tandem: 1st report. Bulletin of the Japan Society of Mechanical Engineers, 24
(188), 323-331.
Jee, M.J.,
Hughes, J.P., Menanteau, F., Sifon, C., Mandelbaum, R., Barrientos, L.F.,
Infante, L. and Ng, K.Y. 2014. Weighing “El Gordo” With a Precision Scale:
Hubble Space Telescope Weak-Lensing Analysis of the Merging Galaxy Cluster
Act-Cl J0102–4915 At z= 0.87. The Astrophysical Journal, 785(1),
p.20.
Johnson, A.A.
and Tezduyar, T.E., 1996. Simulation of multiple spheres falling in a
liquid-filled tube. Computer Methods in Applied Mechanics and
Engineering, 134(3-4),351-373.
Kyle CR. 1979. Reduction of wind
resistance and power output of racing cyclists and runners travelling in
groups. Ergonomics (22) 387-397
Kim, S.Y., Peter, A.H. and
Wittman, D., 2017. In the wake of dark giants: new signatures of dark matter
self-interactions in equal-mass mergers of galaxy clusters. Monthly Notices
of the Royal Astronomical Society, 469(2),1414-1444.
Lammerzahl, C.,
Preuss, O. and Dittus, H., 2008. Is the physics within the Solar system really understood? Astrophysics
and space science library, 349, p.75.
Liao, C.C.,
Hsiao, W.W., Lin, T.Y. and Lin, C.A., 2015. Simulations of two
sedimenting-interacting spheres with different sizes and initial configurations
using immersed boundary method. Computational Mechanics, 55(6),
pp.1191-1200.
Markevitch, M.,
Gonzalez, A.H., David, L., Vikhlinin, A., Murray, S., Forman, W., Jones, C. and
Tucker, W., 2002. A Textbook Example of a Bow Shock in the Merging Galaxy
Cluster 1E 0657–56. The Astrophysical Journal Letters, 567(1),
p.L27.
Markevitch, M.,
Gonzalez, A.H., Clowe, D., Vikhlinin, A., Forman, W., Jones, C., Murray, S. and
Tucker, W., 2004. Direct constraints on the dark matter self-interaction cross
section from the merging galaxy cluster 1E 0657–56. The Astrophysical
Journal, 606(2), p.819.
McCole, S.D.,
Claney, K., Conte, J.C., Anderson, R. and Hagberg, J.M., 1990. Energy
expenditure during bicycling. Journal of Applied Physiology, 68(2),
pp.748-753.
Milgrom, M.,
2014. MOND theory. Canadian Journal of Physics, 93(2),
pp.107-118.
Nielsen, J.T.,
Guffanti, A. and Sarkar, S., 2016. Marginal evidence for cosmic acceleration
from Type Ia supernovae. Scientific reports, 6,
p.35596.
Olds, T. 1998.
The mathematics of breaking away and chasing in cycling. European
journal of applied physiology and occupational physiology, 77(6),
492-497.
Rácz, G., Dobos, L., Beck, R., Szapudi, I. and
Csabai, I., 2017. Concordance cosmology without dark energy. Monthly
Notices of the Royal Astronomical Society: Letters, 469(1), pp.
L1-L5. [“separate universe theory noted, with differences in expansion rates in
different regions of space]
Riess, A.G.,
Filippenko, A.V., Challis, P., Clocchiatti, A., Diercks, A., Garnavich, P.M.,
Gilliland, R.L., Hogan, C.J., Jha, S., Kirshner, R.P. and Leibundgut,
B.R.U.N.O., 1998. Observational evidence from supernovae for an accelerating
universe and a cosmological constant. The Astronomical Journal, 116(3),
p.1009.
Rubin, V.C.,
1983a. The rotation of spiral galaxies. Science, 220(4604),
pp.1339-1344.
Sanders, R.H.,
2014. A historical perspective on modified Newtonian dynamics. Canadian
Journal of Physics, 93(2), pp.126-138.
Scarpa, R.,
2006, March. Modified newtonian dynamics, an introductory review. In AIP
Conference Proceedings (Vol. 822, No. 1, pp. 253-265). AIP.
Sofue,
Y. & Rubin, V. 2001. Rotation Curves of Spiral Galaxies. ARA&A 39,
137–174.
Tchieu, A.A.,
Crowdy, D. and Leonard, A., 2010. Fluid-structure interaction of two bodies in
an inviscid fluid. Physics of Fluids, 22(10), p.107101.
Trenchard, H.
and Perc, M., 2016. Energy saving mechanisms, collective behavior and the
variation range hypothesis in biological systems: A review. BioSystems, 147,
pp.40-66.
Tian, S., Cao, S. and Zhu, Z.H., 2017. The
Dynamics of Inhomogeneous Dark Energy. The Astrophysical Journal, 841(1),
p.63.
Verlinde, E., 2017. Emergent
gravity and the dark universe. SciPost Physics, 2(3), p.016.
Wang, L., Guo,
Z.L. and Mi, J.C., 2014. Drafting, kissing and tumbling process of two
particles with different sizes. Computers & Fluids, 96,
pp.20-34.
Images
http://www.dailymail.co.uk/sport/article-2559386/Sport-images-day-Our-picture-editors-picks-February-14.html (single file mixed)
http://img.rtve.es/v/1511147/ (echelons)
https://www.pinterest.com/pin/378865387381994136/ (single file)
http://d1y1lc6vjc8q07.cloudfront.net/wp-content/uploads/2014/10/0276-300x400.png
http://res.cloudinary.com/steven-cheng/image/upload/v1456470385/calculation_0003_cpohrx.png (elliptical cone)
http://chandra.harvard.edu/photo/2006/1e0657/1e0657_4k.jpg (bullet cluster)
http://www.astro.caltech.edu/~aam/science/thesis/total/node20.html (King profile factors)
brightness, core radius, tidal radius
http://wwwmpa.mpa-garching.mpg.de/galform/virgo/millennium/
https://www.space.com/31926-galaxy-clusters-dark-matter-complicated-relationship.html





















No comments:
Post a Comment